One of the most fundamental properties of the integral is usually presented as follows: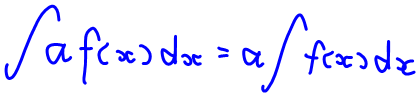
This means that multiplying by a constant before doing the integral is the same as doing the integral and then multiplying by a constant. However, the way it’s presented here makes it look like a rule for algebraic manipulation – I can move a constant multiple in and out of the integral sign. I do actually use it this way when I want to do algebraic manipulation — it comes in handy when I’m creating a reduction formula, for example. But most of the time when I do an integral, I don’t use it that way at all.
Many of my students do use it exactly as it’s presented when they do integrals. Their working will look something like this:
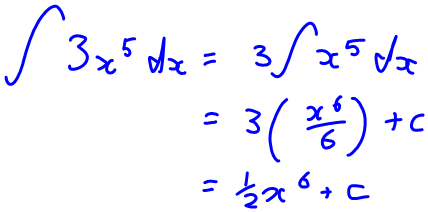
To me, it just seems clunky. Perhaps it’s because the integral sign is so very big and so two of them takes up so much mental space. Or perhaps it feels like we’re waiting around for something good to happen. Anyway, I don’t like it. My working looks something like this:

My thinking on that first line uses the following mantra:

I think, “The 3 is multiplied on so it will stay there”, and I write the “3 times”. Then I think, “to do the integral of x2, I need to put the power up by one and divide by the new power”, and I write that down. Then of course I put the +C.
I find this approach simplifies my thinking about integrals a lot. When I’m doing, say, integration by substitution, I don’t have to move constants in and out of the integral sign. I just leave the constant there, because constants multiplied on stay there. When I’m doing integration by parts, I don’t need to move the numbers out the way in order to focus. I just leave them there because constants multiplied on stay there.
It also simplifies my thinking with derivatives. If there was a constant outside of a bracket, I used to have to expand it out in order to see what to do with the derivative. Now I just say “constants multiplied stay there”, write down the constant and the brackets, and happily do the derivative inside the brackets. It makes me particularly happy when doing partial derivatives: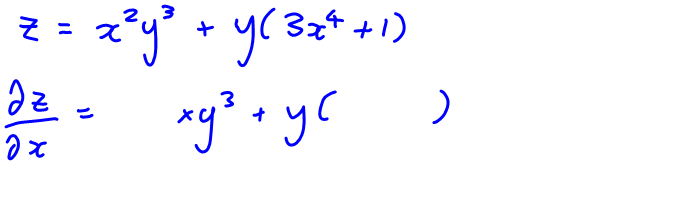

I do need to be a bit careful doing this with students, because it’s qualitatively different to their approach. A lot of the time, when one-on-one with a student, I won’t bring it up and will just use whatever approach they have already shown me in their working so far. When I do use mine, I remember to be even more explicit than usual about my thought process.
In the end, it works for me, so I’ll keep using it at least for myself. It it appeals to you, please do try it and see if it changes your thinking about doing derivatives and integrals too.
