This is the last (for now) in a series of posts about Where the Complex Points Are. To catch you up, I discovered a way of visualising where the complex points are in relation to the points of the real plane. All the complex points (p+ri,q+si) are in a plane attached to the real plane at the point (p,q). I call these planes the “iplanes”, and I imagine it as a transparent sheet that can be unfolded to lie flat on the real plane in order to see its complex points. Using this model, I’ve investigated where the complex points are on a line, on a parabola, and on the graph of a complex function.

Here are links to all the other posts in the series:

• Where the complex points are: introduction to the iplane concept
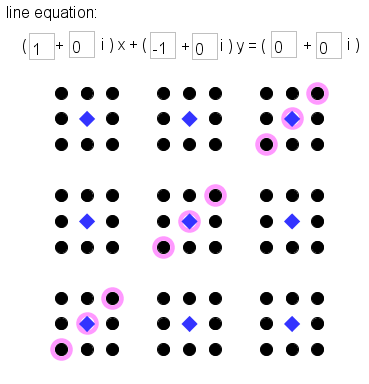
• Where the complex points are: on a line
• Where the complex points are: on a parabola
• Where the complex points are: the graph of a function

There is one more thing I want to talk about and that’s where the idea came from, because I think it’s an interesting story and I want it recorded somewhere.

Between not beyond

Ever since I first learned about complex solutions to quadratic equations, I have wondered where the complex points are. I never really thought about it deeply, but the general picture in my head was that the complex points were somehow “beyond” the real plane. I imagined them as over there beyond the edge of the real plane, surrounding it so that the real plane was a small plane inside some much bigger plane.

But something doesn’t quite work about this picture. When I studied conics in depth much much later I learned that a “degenerate circle” with equation x² + y² = 0 wasn’t just the origin (0,0) as I had originally thought. In fact it was a pair of complex lines that each happen to have one real point. (These lines have equations x+iy=0 and x-iy=0.)

Only recently did this start to bother me. Suddenly I asked myself, if the complex points are beyond the real plane, how can a line made almost entirely of complex points meet the real plane in the origin, right there in the centre?

It occurred to me that the line must somehow travel over or between the real points to arrive at the origin in the middle.

And the seed of an idea began to grow. If the lines have to travel between the other points to reach the origin, then the complex points must somehow be bewteen the real points, not beyond them.

Finite planes – the smallest plane

Once I realised that the complex points had to be between the other points, I started thinking about the problem in terms of finite planes. In a finite geometry, the points are discrete points, and there is plenty of space between them to put extra points, if indeed that’s where they are.

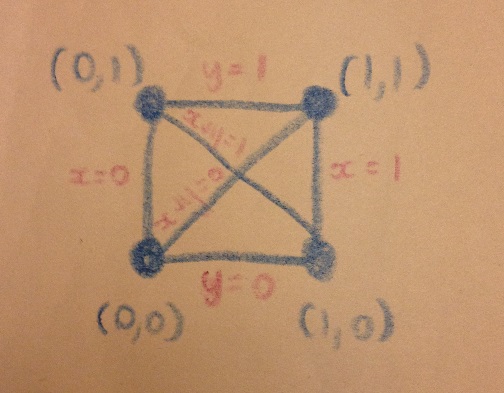
I started out with the smallest possible affine plane (our ordinary geometry is a kind of affine plane). It has four points with coordinates taken from the set {0,1} (also known as Z₂) and six lines. The lines have equations x=0, x=1, y=0, y=1, x+y=0, x+y=1. One name for this plane is AG(2,2) – the Affine Geometry of dimension 2 and order 2.

What I needed was to find where the “complex points” were in relation to this plane. Unfortunately, if you’re using Z₂, 1+1=0 so -1 is the same as 1. This means the equation x² = -1 already has a solution, so I can’t add the number i. You actually have to add the solution to x² + x = 1. I called this number α so that I ended up with a set of four numbers to use for coordinates: {0,1,α, 1+α}.

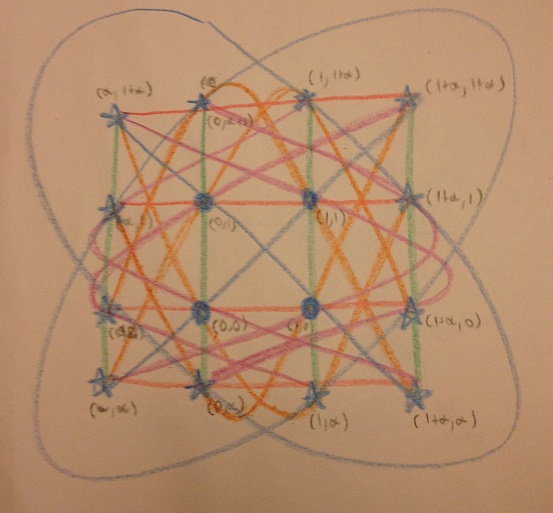
From this I can build AG(2,4), which has 16 total points, and 20 total lines. I wrote down the equations of all the new lines and tried to figure out how to add the points to my diagram so that the new lines looked like lines.

I knew the lines in my new plane would have to have four points — one for each “number” in the coordinates — and this meant each line already existing needed two extra points. It seemed reasonable to exend them outwards. It also seemed reasonable to try and make the new lines x=α and x=1+α vertical and the new lines y=α and y=1+α horizontal.

Fiddling around with this gave me a nice square arrangement for the points. Then I investigated what all the new lines looked like and I got something like this. (Note I was drawing in crayon on the paper tablecloths at my sister’s restaurant at the time. I didn’t take a photo on the day, but this is a reasonable facsimile.) You can see the lines a little more clearly in this GeoGebra applet.

Unfortunately the extra points were outside the original ones, and not between them as I had hoped. I did notice that each new line that wasn’t part of the original plane met the original plane in exactly one point. I knew this would have to happen because it’s a theorem from finite geometry, but I was glad that everything was working the way it should.

Finite planes — noughts and crosses saves the day!

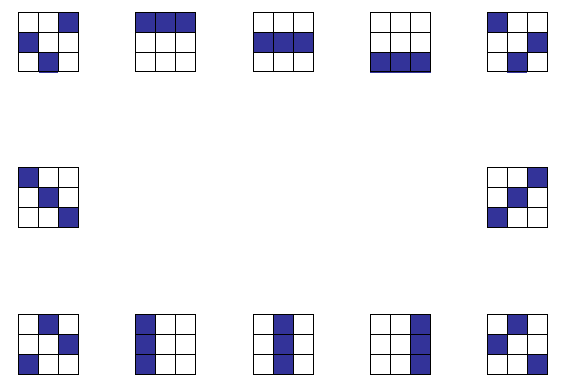
After the half-success of the smallest affine plane, it was natural to move on to the second-smallest affine plane AG(2,3). This is the plane you get when you add in all the wraparound diagonals to a Noughts and Crosses board. When drawing it on a noughts-and-crosses board, the twelve lines look like this:

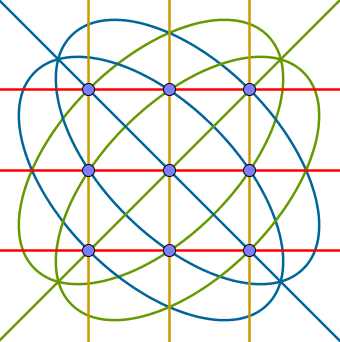
If you draw the points as dots and the lines as (curved) lines, you can make it look like this. You can see the individual lines more clearly in this GeoGebra applet.

As you can see, it’s already quite invovled, and I didn’t really like to imagine what would happen when I went up to the “complex” plane — that would mean going from 9 points to 81 points and from 12 lines to 90 lines. The 20 lines of the affine plane of order 4 were enough of a mess!

But I did start to investigate. AG(2,3) has its coordinates taken from Z₃, which is {-1,0,1} with the rule that 1+1=-1. This field doesn’t have any solutions to x²=-1, so it was safe to attach the number i this time. I drew my extra points clustered around the existing points and started to investigate the lines I would need. In order to get the lines with equations like x=i to be vertical, I needed to make sure that the points with coordinates (i,something) were lined up above each other. This required me to make sure that within each cluster of points the same imaginary parts were added to the coordinates.

Here’s a picture of my ideas book around this time:

After drawing it on paper, it was time to investigate it more fully, so I worked on creating a GeoGebra applet that would show the points on a specific complex line. It took some fancy footwork with the spreadsheet to make it do what I wanted.

I noticed now that the extra points on every real line lined up neatly with the real line. This was totally awesome, and I was inspired to move on to the real plane to see what was happening there.

Finally iplanes are found

I was all satisfied with how my complex points worked in finite planes. The final step was to think about how this applied to the infinite real plane.

There was a big problem, of course. In the finite planes, there was plenty of space between the points to fit the extra points. In the contiuous real plane we’re already full. Where do I fit those extra points?

My first idea was that they were all squished into each point of the plane — “fat” points, as it were. But this was somehow unsatisfying because I couldn’t actually see where the points were. Then it occurred to me that if I couldn’t squish them into each point, they’d have to be above the plane but attached at the real point. And the iplane was born.

At the start, I was sketching and calculating on scrap paper, drawing a little square around the real point to visualise the iplane. This was giving me enough insight to get a feel for what was going on with lines. The GeoGebra applets in the previous posts are based on this visualisation. It wasn’t until I got out the cellophane and the tap-tap board that I really felt like I had a proper feel for what was going on. This pinkness has transferred to all of my diagrams and all of my thinking about iplanes, but you’ve seen the results of all that thinking already…

Conclusion

So what is the point of this story? I don’t know. I just thought you (and future me) might like to see my train of thought in creating this idea.

I suppose for me the main moral is that finite geometry helps give me insights into real geometry, and that my habit of making physical models of things is still a worthwhile thing to foster.