I have a whole suite of maths t-shirts that I made myself. One of them simply has the number 65536 on it. It’s been getting a bit of attention over the past couple of weeks, so I thought I might write about it.
65536 is my favourite power of 2. More specifically, it’s 216, which means you can make it by starting at 1 and multiplying by 2 sixteen times. Even better…
But this cool stack of powers is not why it’s my favourite powet of 2. It’s my favourite power of two because of its connection to two very cool ideas in maths.
Firstly, 65536 is the last known power of 2 for which the next number is prime. It’s known that if a number one more than a power of 2 is a prime then it must be 2^(2^n)+1 for some n. The first five are all prime
- 2^(2^0)) +1 = 3
- 2^(2^1)) +1 = 5
- 2^(2^2)) +1 = 17
- 2^(2^3)) +1 = 257
- 2^(2^4)) +1 = 65537
Fermat apparently conjectured in 1650 that they were all prime, which is why numbers of the form 2^(2^n)+1 are called “Fermat numbers” and if they’re prime they’re called “Fermat primes”. But so far, no more Fermat primes have been found. That is, every bigger number of the form 2^(2^n)+1 that we can calculate has been found to not be prime after all. Yet we haven’t been able to prove that there are definitely no more of them.
Isn’t that amazing? In close to 400 years we haven’t been able to find any more Fermat primes, but neither have we been convinced beyond a doubt that there aren’t any. I think it’s awesome that in maths there are things so simple that at the moment are unknown.
Secondly, 65536 is the only known power of 2 with no powers of two in order among its digits. Every other power of two where we have a list of most its digits, you can cross out some of the digits and have a power of two left behind. But we just don’t know if somewhere out there there’s a really big power of 2 that again lacks any smaller powers of two among its digits. Even stronger than this, every other power of two we’ve calculated has a 1, 2, 4, or 8 among its digits, but again we don’t know if somewhere out there in the distance there might be one that lacks these four digits.
What makes this surprising is that there is a perfectly good pattern to the final digits of the powers of 2. The last digit goes in the pattern 2, 4, 8, 6, 2, 4, 8, 6, … and the last two digits go in the pattern 04, 08, 16, 32, 64, 28, 56, 12, 24, 48, 96, 92, 84, 68, 36, 72, 44, 88, 76, 52, 04, … You’d think that the repeating pattern of the final digits might make it easy to tell what digits were in a power of 2, but it’s not nearly so easy.
What’s even more surprising is that the same concept for prime numbers is completely solved. If you cross out some of the digits of a prime number you might have a prime number left behind. For example, the prime number 16649 leaves behind the prime number 19 when you cross out the 664. So which prime numbers have no prime numbers among their digits? Well there’s exactly 26 of them and they are 2, 3, 5, 7, 11, 19, 41, 61, 89, 409, 449, 499, 881, 991, 6469, 6949, 9001, 9049, 9649, 9949, 60649, 666649, 946669, 60000049, 66000049, 66600049. That’s it. All of them. Every other prime number has at least one of these in order among its digits.
This is from a paper in 2000 from Jeffrey Shallit: “Minimal primes,” J. Recreational Math., 30:2 (1999–2000) 113–117. He talks about it here. Within a set of numbers, he calls the “minimial set” those ones with none of the others in the set in order among their digits. He references another author’s theorem which says that given any set of numbers, the minimal set within it must be finite.
Isn’t it amazing that the prime numbers with all their apparent randomness have allowed us to find their minimal set, but the powers of two with their obvious regularity haven’t?
So that’s why 65536 is my favourite power of 2. It represents to me some cool ideas, and more than that, it reminds me that maths is far from all done in the distant past, it’s got unanswered questions alive right now.


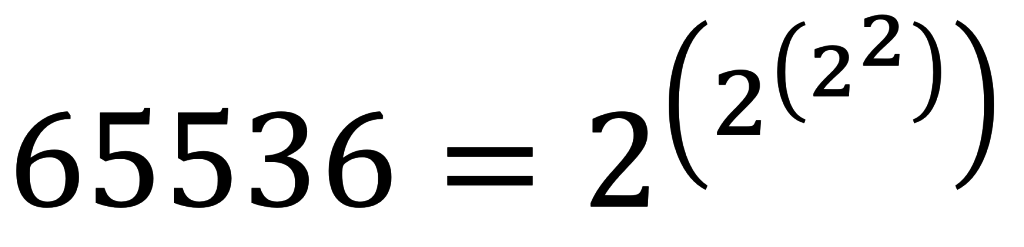
I normally expect to learn one or two things from a good blog post but I feel like I’ve just learned fifteen. I have a new found appreciation for the number 65536.
apm
Twitter: @autismplusmath
So glad it made a difference to you!
[…] The first is about why 65536 is his favorite power of 2. […]
[…] is 2⁸ + 1 and is the second largest known Fermat Prime. Click that link to see how unusual and cool Fermat Primes […]
Fun that 65536+1 is easy to remember as the 6543rd prime:). The 654321th prime-1 isn’t as cool, apart from being 3^2*2^2*273131 .
What a fun Saturday morning read!
Glad you liked it!