This blog post is about a new variation on the classic Quarter the Cross problem, which I call Quarter the Cross: Connect the Dots.
Background
Here is the original Quarter the Cross problem:
To catch you up, here is everything I’ve written about Quarter the Cross up until now:
- Quarter the Cross — in which I first learn about Quarter the Cross and become thoroughly obsessed, making 100 solutions to the problem.
- A Day of Maths 2: Quarter the Cross — in which I bring Quarter the Cross into my daughter’s Year 7 classroom.
- David Butler and the Prisoner of Alhazen — in which I become a prisoner of the awesome Lunes of Alhazen via Quarter the Cross
- Quarter the Cross: Colouring — in which I draw All. The. Lines. and so create a colouring template for Quarter the Cross
Even without reading those posts, you can probably infer that I really love Quarter the Cross. And you’d be right. I love how you have to think a bit hard to get any solution, but once you get started there is so much freedom to be creative.
But sometimes, you feel like you don’t want quite so much room for creativity. You want some more constraints so you don’t feel awash in the entire universe of possibilities, most of which you can’t even think of. Alternatively, you might enjoy the creativity but you are running a bit dry and need some more constraints to push you to try new things. Finally, Quarter the Cross might seem all a bit familiar to you, and you still want to play, but you need something to make it new. This new version of Quarter the Cross provides a solution to all of these problems.
A new constraint: connect the dots
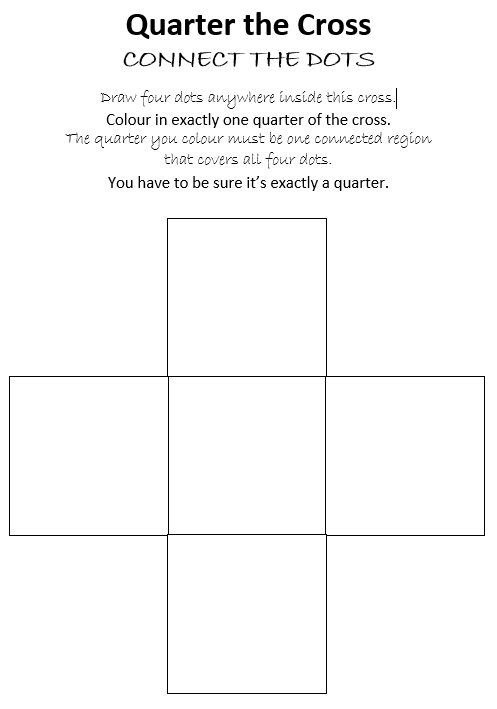
Here is the new version of the puzzle, to use when you feel the need for an extra constraint.
(A downloadable Word document with this cross made of 3cm squares and the instructions is here.)
This Connect the Dots version is an easy way to turn the original Quarter the Cross into a new challenge. One bonus feature is that someone else can put the dots in for you, making it more of a surprise. If you would like a computer to choose for you, I made this Desmos graph. Note that you could choose a different number of dots than four (and the Desmos graph allows you to do so) but I find it’s about the right number to make the challenge easy to set up and not too annoying to do.
I personally very much enjoyed this challenge. It forced me to think in new ways, because I couldn’t just put the shapes I would normally use wherever I wanted. I had to do a lot more thinking about how pieces added up to a quarter because I had to stretch them out to meet each other. I also had to let go of an attachment to symmetry. (Though I now realise it could have been an extra extra challenge to make the solution symmetrical in some way as well as connect the dots!)
I’ll finish with some tweets with solutions to Quarter the Cross: Connect the Dots challenges. If you want to try the challenge yourself before seeing others’ solutions, please look away now! Either way, I hope you enjoy this variation on a classic.
I am really surprised by how hard this #QuarterTheCross dot challenge is, especially when you look for a connected region. It’s a really different kind of thinking. pic.twitter.com/4nwW4cLaia
— David Butler (@DavidKButlerUoA) May 24, 2018
An alternative solution to this #QuarterTheCross dot challenge: pic.twitter.com/auuQBzo7YE
— David Butler (@DavidKButlerUoA) May 24, 2018
One more Connect the Dots #QuarterTheCross from @JeremyInSTEM at #100factorial today. pic.twitter.com/8Z6BMm8RLQ
— David Butler (@DavidKButlerUoA) May 30, 2018